A Regular Cosine Series with Odd $k$

Consider the Fourier series of the unit-amplitude triangular wave. As is well known it is given by the cosine series

$$S_{\rm c} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, \cos[(2j+1)\theta].$$

The corresponding FC series is then

$$\bar{S}_{\rm c} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, \sin[(2j+1)\theta],$$

the complex $S_{v}$ series is given by

$$S_{v} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, v^{2j+1},$$

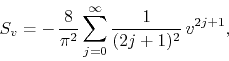
and the complex power series $S_{z}$ is given by

$$S_{z} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, z^{2j+1}.$$

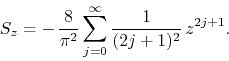
The ratio test tells us that the disk of convergence of $S_{z}$ is the unit disk. If we consider the inner analytic function $w(z)$ within this disk we observe that $w(0)=0$, as expected. We have for the function and its derivative

$$\begin{eqnarray*} w(z) & = & -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \fr... ...frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{2j+1}\, z^{2j}. \end{eqnarray*}$$

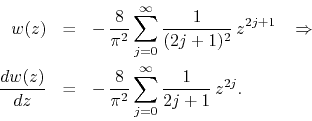
Observe that we have for the derivative the particular value

$$\frac{dw}{dz}(0) = -\, \frac{8}{\pi^{2}}.$$

We may now multiply by $z$ and differentiate again, to obtain

$$\begin{eqnarray*} \frac{d}{dz} \left[ z\, \frac{dw(z)}{dz} \right] & = & ... ...\\ & = & -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} z^{2j}. \end{eqnarray*}$$

This is a geometrical series and therefore we may write this expression in closed form,

$$\begin{eqnarray*} \frac{d}{dz} \left[ z\, \frac{dw(z)}{dz} \right] & = & ... ...8}{\pi^{2}}\, \left( \frac{1}{1+z} + \frac{1}{1-z} \right). \end{eqnarray*}$$

It is now very simple to integrate in order to obtain the derivative of $w(z)$ in closed form, remembering that we should have for it the value $-8/\pi^{2}$ at $z=0$,

$$\begin{eqnarray*} z\, \frac{dw(z)}{dz} & = & -\, \frac{4}{\pi^{2}}\, \left... ... & -\, \frac{4}{\pi^{2}}\, \ln\!\left(\frac{1+z}{1-z}\right). \end{eqnarray*}$$

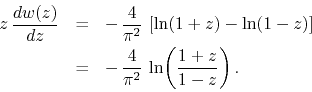
This is the logarithmic derivative of $w(z)$, which is another inner analytic function, and it should be noted that it is proportional to the analytic function $w_{\rm sq}(z)$ for the case of the standard square wave,

$$z\, \frac{dw(z)}{dz} = -\, \frac{2}{\pi}\, w_{\rm sq}(z).$$

We get for the derivative of $w(z)$

$$\frac{dw(z)}{dz} = -\, \frac{4}{\pi^{2}}\, \frac{1}{z}\, \ln\!\left(\frac{1+z}{1-z}\right).$$

This function has two borderline hard singularities at the points where the triangular wave is not differentiable. Presumably $w(z)$ has two borderline soft singularities at these points. It seems that the second integration cannot be done explicitly because the indefinite integral of the function above cannot be expressed as a finite combination of elementary functions. We are thus unable to write $w(z)$ in closed form. However, we may still obtain partial confirmation of our results by using the closed form for the derivative of $w(z)$. If we differentiate the Fourier series of the triangular wave with respect to $\theta$ we get

$$\frac{dS_{\rm c}}{d\theta} = \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{2j+1}\, \sin[(2j+1)\theta].$$

This is equal to $2/\pi$ times the Fourier series for the standard square wave, given in Subsection C.2. On the other hand, if we use $z=\rho\exp(\mbox{\boldmath$\imath$}\theta)$ and consider the complex derivative of $w(z)$ taken in the direction of $\theta$, we have

$$\frac{dw(z)}{d\theta} = \mbox{\boldmath$\imath$} z\, \frac{dw(z)}{dz}.$$

We have therefore

$$\begin{eqnarray*} \frac{dw(z)}{d\theta} & = & -\mbox{\boldmath$\imath$}\, \f... ... & -\mbox{\boldmath$\imath$}\, \frac{2}{\pi}\, w_{\rm sq}(z). \end{eqnarray*}$$

The factor of $-\mbox{\boldmath$\imath$}$ simply implements the necessary exchanges of real and imaginary parts, to account for the exchange of sines and cosines in the process of differentiation, and the factor of $2/\pi$ corrects the normalization. We see therefore that at least the derivative of the Fourier series at the unit circle is represented correctly by the inner analytic function $w(z)$.