Next: Final Comments Up: On the Convergence of Previous: Possible Algorithmic Applications
It is interesting that one may establish without too much difficulty at least one analytical property of the functions to which the class of Fourier series studied here converge, which is related to their continuity over the unit circle. For simplicity, let us limit this discussion to the simplest type of series, the one which we discussed in detail in this paper. However, there should be no difficulty in extending the ideas to the generalizations mentioned before.
First let us observe that, for series of bounded functions such as the
complex basis
![]() , for which the modulus of each
function is unity, and hence independent of the variable
, for which the modulus of each
function is unity, and hence independent of the variable ![]() ,
absolute convergence implies convergence with criteria which do not depend
on the variable
,
absolute convergence implies convergence with criteria which do not depend
on the variable ![]() , and therefore imply uniform convergence. This
can also be established by the use of the Weierstrass
, and therefore imply uniform convergence. This
can also be established by the use of the Weierstrass ![]() -test. Hence,
given any closed interval of the unit circle which does not contain the
special point
-test. Hence,
given any closed interval of the unit circle which does not contain the
special point ![]() , for which
, for which ![]() , the series in the following
expression for
, the series in the following
expression for ![]() ,
,
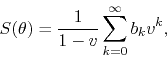
is uniformly convergent within that interval. Therefore, using the
well-known fact that a series of continuous functions which converges
uniformly over a closed interval does so to a continuous function, it
follows that the series converges to a continuous function within that
interval. This implies that the whole class of Fourier series discussed
here, in this simplest case, converge to continuous functions, everywhere
except for the special point ![]() over the unit circle. It is clear
that similar properties hold for the extensions of the theorem in which
there are several such special points.
over the unit circle. It is clear
that similar properties hold for the extensions of the theorem in which
there are several such special points.
However, the differentiability properties of these functions are not so
easily determined. Since the series in the right-hand side is absolutely
and hence uniformly convergent, it may be possible to differentiate it
term-by-term to produce a convergent series, even if one certainly cannot
do the same to the original series for ![]() , when that series is
not absolutely convergent. Using the fact that
, when that series is
not absolutely convergent. Using the fact that
we may attempt to calculate the derivative of ![]() , thus obtaining
, thus obtaining
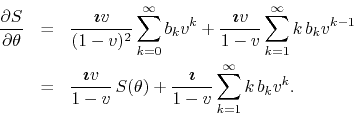
The series contained within ![]() in the first term is convergent
due to our theorem, so the differentiability of the function is related to
the convergence or lack of convergence of the series in the second term,
which depends on the behavior of the coefficients
in the first term is convergent
due to our theorem, so the differentiability of the function is related to
the convergence or lack of convergence of the series in the second term,
which depends on the behavior of the coefficients
![]() . If
these coefficients turn out to be such that the corresponding series is
absolutely convergent within the closed interval mentioned before, then it
follows that the function is differentiable in that interval.
. If
these coefficients turn out to be such that the corresponding series is
absolutely convergent within the closed interval mentioned before, then it
follows that the function is differentiable in that interval.
Absolute convergence depends on the coefficients ![]() going to zero
sufficiently fast with
going to zero
sufficiently fast with ![]() , while term-by-term differentiation implies in
a factor of
, while term-by-term differentiation implies in
a factor of ![]() multiplying
multiplying ![]() . Therefore, while it is possible that
some of these functions are continuous, it seems likely that many are not.
In fact, since the original series defining
. Therefore, while it is possible that
some of these functions are continuous, it seems likely that many are not.
In fact, since the original series defining ![]() may converge very
slowly and may contain significant Fourier components for very large
frequencies, it does lead to the impression that the limiting functions
might not be differentiable at all, undergoing violent high-frequency
oscillations everywhere. At first glance it certainly seems very unlikely
that these functions may have more than the first derivative, due to the
increasing powers of
may converge very
slowly and may contain significant Fourier components for very large
frequencies, it does lead to the impression that the limiting functions
might not be differentiable at all, undergoing violent high-frequency
oscillations everywhere. At first glance it certainly seems very unlikely
that these functions may have more than the first derivative, due to the
increasing powers of ![]() multiplying
multiplying ![]() , which are implied by
multiple term-by-term differentiation.
, which are implied by
multiple term-by-term differentiation.
However, there is another possibility, namely that the coefficients
![]() turn out to also satisfy the hypotheses of our theorem. In this
case one would be able to transform the corresponding series once more
into one which is absolutely convergent, which would then imply the
differentiability of the function. For cases in which this procedure can
be iterated, it might be possible to show that the functions also have the
second derivative, or even higher-order ones. The crucial hypothesis is
that of the monotonicity of
turn out to also satisfy the hypotheses of our theorem. In this
case one would be able to transform the corresponding series once more
into one which is absolutely convergent, which would then imply the
differentiability of the function. For cases in which this procedure can
be iterated, it might be possible to show that the functions also have the
second derivative, or even higher-order ones. The crucial hypothesis is
that of the monotonicity of ![]() , and that depends essentially on the
monotonicity of
, and that depends essentially on the
monotonicity of ![]() . If there are series for which the transformation
from
. If there are series for which the transformation
from ![]() to
to ![]() preserves the monotonic character of the sequence,
then these series should converge to functions which are differentiable in
the closed interval mentioned above.
preserves the monotonic character of the sequence,
then these series should converge to functions which are differentiable in
the closed interval mentioned above.
Conceivably, there might even exist series for which this procedure of the
transformation of the coefficients can be iterated indefinitely. In such a
case the limiting functions would turn out to be infinitely differentiable
within the closed interval, that is, of class
![]() . We must
conclude, therefore, that the differentiability properties of these
functions remain as completely open questions.
. We must
conclude, therefore, that the differentiability properties of these
functions remain as completely open questions.