Invariance of Definite Integrals

Let us determine the effect of the filter on the definite integral of a function $f(x)$ with compact support on the real line. We may write the integral as

$$I = \int_{-\infty}^{\infty}dx\, f(x),$$

where the integrand is non-zero only inside a closed interval. The integral of the filtered function $f_{\varepsilon}(x)$ has support on another closed interval, that of $f(x)$ increased by $\varepsilon$ in each direction, and is similarly given by

$$\begin{eqnarray*} I_{\varepsilon} & = & \int_{-\infty}^{\infty}dx\, f_{\vare... ... \int_{x-\varepsilon}^{x+\varepsilon}dx'\, f\!\left(x'\right), \end{eqnarray*}$$

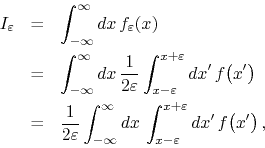
where we used the definition of $f_{\varepsilon}(x)$ in terms of $f(x)$. We now make the change of variables $x'=x''+x$ on the inner integral, implying $dx'=dx''$ and $x''=x'-x$, leading to

$$\begin{eqnarray*} I_{\varepsilon} & = & \frac{1}{2\varepsilon} \int_{-\infty... ...lon}^{\varepsilon}dx''\, \int_{-\infty}^{\infty}dx\, f(x''+x). \end{eqnarray*}$$

Since the integral on $x$ is over the whole real line, we may now change variables on it without changing the integration limits, using $x=x'-x''$, with $x'=x''+x$ and $dx=dx'$, and thus obtaining

$$\begin{eqnarray*} I_{\varepsilon} & = & \frac{1}{2\varepsilon} \int_{-\varep... ...lon} \int_{-\varepsilon}^{\varepsilon}dx''\, I \\ & = & I, \end{eqnarray*}$$

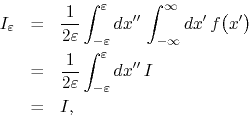
were we recognized the form of the integral $I$. In this way we show that $I_{\varepsilon}=I$, that is, the filter does not change the definite integral at all. Another way to state this is to say that the filter does not change the average value of $f(x)$ over the common support of $f(x)$ and $f_{\varepsilon}(x)$.