Points of Discontinuity

Let us show that in the $\varepsilon\to 0$ limit the function $f_{\varepsilon}(x)$ essentially reproduces the original function $f(x)$. Stating it more precisely, we will show that, if the function $f(x)$ has an isolated point of discontinuity at $x_{0}$, then in the $\varepsilon\to 0$ limit $f_{\varepsilon}(x_{0})$ tends to the average of the two lateral limits of $f(x)$ to the point $x_{0}$, that is,

$$\lim_{\varepsilon\to 0}f_{\varepsilon}(x_{0}) = \frac{1}{2}\left({\cal L}_{+}+{\cal L}_{-}\right),$$

where

$${\cal L}_{\pm} = \lim_{x\to x_{0\pm}}f(x),$$

regardless of the value that $f(x)$ assumes at $x_{0}$. In particular, if $f(x)$ is continuous at $x_{0}$, then ${\cal L}_{+}={\cal L}_{-}=f(x_{0})$ and hence $f_{\varepsilon}(x_{0})$ tends to $f(x_{0})$ in the limit, thus reproducing the original function at that point.

Here is the proof: if $f(x)$ has an isolated point of discontinuity at $x_{0}$, then there are two neighborhoods of $x_{0}$, one to the left and another one to the right, where $f(x)$ is continuous. For sufficiently small $\varepsilon$, the interval of integration will fit into this combined neighborhood, so that the only point of discontinuity within it will be $x_{0}$. Let us consider then the value of $f_{\varepsilon}(x_{0})$, as given by the definition,

$$f_{\varepsilon}(x_{0}) = \frac{1}{2\varepsilon} \int_{x_{0}-\varepsilon}^{x_{0}+\varepsilon}dx'\,f(x').$$

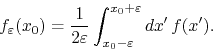
We may separate this integral in two, one in the left neighborhood and another one in the right neighborhood,

$$f_{\varepsilon}(x_{0}) = \frac{1}{2\varepsilon} \int_{x_... ...{1}{2\varepsilon} \int_{x_{0}}^{x_{0}+\varepsilon}dx'\,f(x').$$

Since the function $f(x)$ is integrable, the integrals converge to $\varepsilon$ times the average value of the function over each sub-interval, so that we have

$$\lim_{\varepsilon\to 0}f_{\varepsilon}(x_{0}) = \frac{1}{2} \bar{f}(x_{0-}) + \frac{1}{2} \bar{f}(x_{0+}).$$

Finally, since the function $f(x)$ is continuous in each sub-interval, in the $\varepsilon\to 0$ limit each average value converges to the corresponding lateral limit of $f(x)$, so that we have

$$\lim_{\varepsilon\to 0}f_{\varepsilon}(x_{0}) = \frac{1}{2}\left({\cal L}_{+}+{\cal L}_{-}\right),$$

where

$${\cal L}_{\pm} = \lim_{x\to x_{0\pm}}f(x).$$

This establishes the result. As a consequence of this, if $f(x)$ is continuous at $x_{0}$, then ${\cal L}_{+}={\cal L}_{-}=f(x_{0})$, and therefore we have

$$\lim_{\varepsilon\to 0}f_{\varepsilon}(x_{0}) = f(x_{0}).$$

We therefore conclude that in the $\varepsilon\to 0$ limit the filtered function $f_{\varepsilon}(x)$ reproduces the original function $f(x)$ where it is continuous. There may be isolated points of discontinuity where this fails, and therefore we say that in the $\varepsilon\to 0$ limit the filtered function $f_{\varepsilon}(x)$ reproduces the original function $f(x)$ almost everywhere.