The Expectation Value of $\varphi_{1}'(n_{\mu}')\varphi_{1}'(n_{\mu}'')S_{V}[\vec{\varphi}']$

We now calculate the expectation value

$$\left\langle \varphi_{1}'(n_{\mu}') \varphi_{1}'(n_{\mu}'') S_{V}[\vec{\varphi}'] \right\rangle_{0}.$$

Once more only the field-even part of the action will yield a non-zero result, so that we have

$$\left\langle \varphi_{1}'(n_{\mu}') \varphi_{1}'(n_{\mu}'... ...{\mu}'') S_{V,{\rm even}}[\vec{\varphi}'] \right\rangle_{0}.$$

Using the form of $S_{V,{\rm even}}[\vec{\varphi}']$ shown in Equation (A.3), and if we already replace the expectation values of squared fields by $\sigma_{0}$ or $\sigma_{\mathfrak{N}}$ whenever possible, as well as replace $\left\langle\varphi_{1}'(n_{\mu}')\varphi_{1}'(n_{\mu}'')\right\rangle_{0}$ by $g_{0}(n_{\mu}'-n_{\mu}'')$, we get

$$\begin{eqnarray*} \left\langle \varphi_{1}'(n_{\mu}') \varphi_{1}'(n_{\mu}'')... ..._{\mu}) \right\rangle_{0} g_{0}(n_{\mu}'-n_{\mu}'') \right\}. \end{eqnarray*}$$

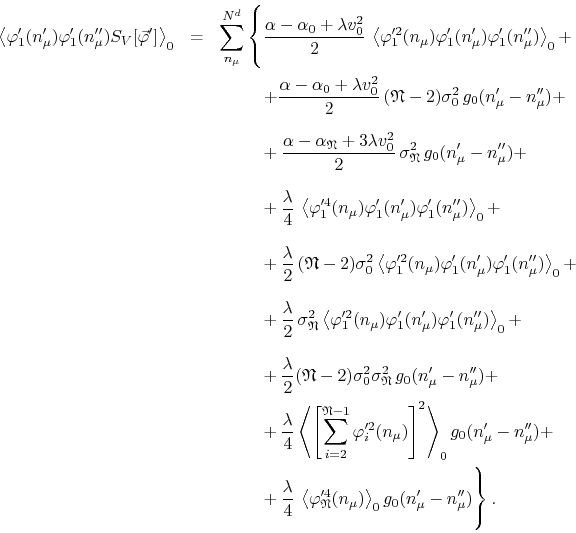
We may now use the known value of the expectation value of the squared sum. From Appendix B, Equation (B.14), we get

$$\left\langle \left[ \sum_{i=2}^{\mathfrak{N}-1} \varphi_... ...t\rangle_{0} = \mathfrak{N}(\mathfrak{N}-2) \sigma_{0}^{4}.$$

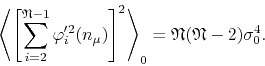
We may also use the fact that it can be shown that

$$\begin{eqnarray*} \left\langle \varphi_{\mathfrak{N}}'^{4}(n_{\mu}) \right\ra... ..._{0}^{2}\, g_{0}(n_{\mu}-n_{\mu}')\, g_{0}(n_{\mu}-n_{\mu}''), \end{eqnarray*}$$

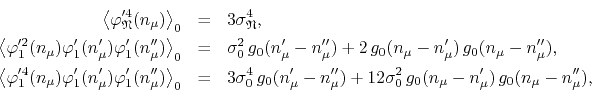
also found in Appendix B, Equations (B.8), (B.10) and (B.12), in order to write for our expectation value

$$\begin{eqnarray*} \left\langle \varphi_{1}'(n_{\mu}') \varphi_{1}'(n_{\mu}'')... ...sigma_{\mathfrak{N}}^{4}\, g_{0}(n_{\mu}'-n_{\mu}'') \right\}. \end{eqnarray*}$$

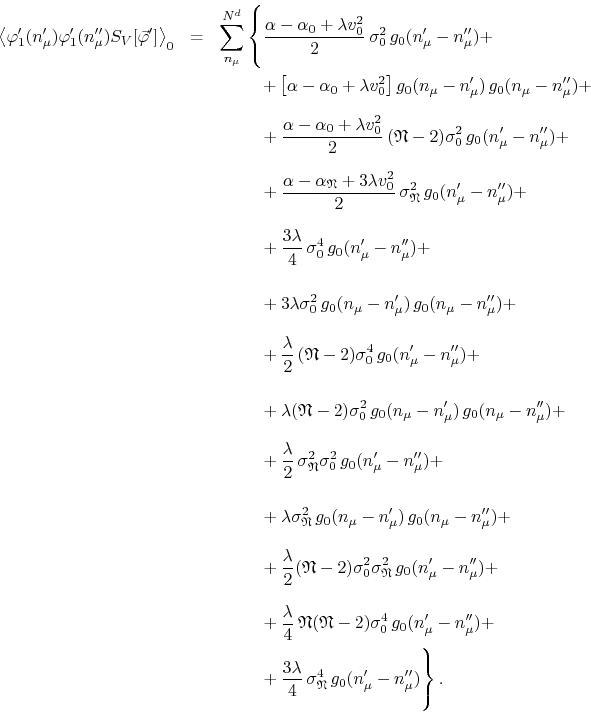
Next we group all terms containing $g_{0}(n_{\mu}'-n_{\mu}'')$ and simplify to get

$$\begin{eqnarray*} \lefteqn { \left\langle \varphi_{1}'(n_{\mu}') \varphi_{1... ... \right\} g_{0}(n_{\mu}-n_{\mu}')\, g_{0}(n_{\mu}-n_{\mu}''). \end{eqnarray*}$$

The sum over $n_{\mu}$ can now be done in all terms in the first group, yielding

$$\begin{eqnarray*} \lefteqn { \left\langle \varphi_{1}'(n_{\mu}') \varphi_{1... ... \right\} g_{0}(n_{\mu}-n_{\mu}')\, g_{0}(n_{\mu}-n_{\mu}''). \end{eqnarray*}$$

We must now perform the sum indicated. This is easily done using Fourier transforms. From Appendix B, Equation (B.4), we get

$$\sum_{n_{\mu}}^{N^{d}} g_{0}(n_{\mu}-n_{\mu}')\, g_{0}(n_... ...mu}'-n_{\mu}'')} } { [\rho^{2}(k_{\mu})+\alpha_{0}]^{2} },$$

which is expressed as a Fourier transform, with the general structure of a two-point function. We have therefore the final result,

$${ \left\langle \varphi_{1}'(n_{\mu}') \varphi_{1}'(n_{\mu}'') S_{V}[\vec{\varphi}'] \right\rangle_{0} }$$

$$= N^{d} \left[ \frac{\alpha-\alpha_{0}+\lambda v_{0}^{2}}{2}\, (\ma... ...pha_{\mathfrak{N}}+3\lambda v_{0}^{2}}{2}\, \sigma_{\mathfrak{N}}^{2} + \right.$$

$$\hspace{2.2em} \left. + \frac{\lambda}{4}\, (\mathfrak{N}^{2}-1) ... ...frac{3\lambda}{4}\, \sigma_{\mathfrak{N}}^{4} \right] g_{0}(n_{\mu}'-n_{\mu}'')$$ (A.6)

$$+ \left[ \alpha-\alpha_{0} + \lambda v_{0}^{2} + \lambda (\mathfr... ...{\mu}^{d}k_{\mu}(n_{\mu}'-n_{\mu}'')} } { [\rho^{2}(k_{\mu})+\alpha_{0}]^{2} }.$$