Monotonicity Extensions

The argument given in the last subsection can be generalized in several ways, and the generalizations constitute proof of the convergence of wider classes of series of type $S_{v,h0}$. For example, it can be trivially generalized to series with negative $a_{k}$ for all $k$, converging to zero from below. It is also trivial that it can be generalized to series which include a non-monotonic initial part, up to some minimum value $k_{m}$ of $k$. In a less trivial, but still simple way, the argument can be generalized to series with only odd-$k$ terms, such as the square wave, or with only even-$k$ terms, such as the two-cycle sawtooth wave. We can prove this in a simple way by reducing these cases to the previous one. If we have a complex power series given by

$$S_{z} = \sum_{j=1}^{\infty} a_{k}z^{k},$$

where $k=2j$, we may simply define new coefficients $a'_{j}=a_{k}$ and a new complex variable $z'=z^{2}$, in terms of which the series can now be written as

$$S_{z} = \sum_{j=1}^{\infty} a'_{j}z^{\prime j},$$

thus reducing it to the previous form, in terms of the variable $z'$. So long as the non-zero coefficients $a_{k}$ tend monotonically to zero as $k\to\infty$, we have that the coefficients $a'_{j}$ tend monotonically to zero as $j\to\infty$, and the previous result applies. The same is true if we have a complex power series given by

$$S_{z} = \sum_{j=0}^{\infty} a_{k}z^{k},$$

where $k=2j+1$, since we may still define new coefficients $a'_{j}=a_{k}$ and the new complex variable $z'=z^{2}$, in terms of which the series can now be written as

$$S_{z} = z \sum_{j=0}^{\infty} a'_{j}z^{\prime j},$$

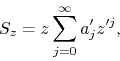
so that once more, so long as the non-zero coefficients tend monotonically to zero, the previous result applies. We say that series such as these have non-zero coefficients with a constant step $2$. The only important difference that comes up here is that the special points on the unit circle are now defined by $z'=1$, which means that $z^{2}=1$ and hence that $z=\pm 1$. Therefore, in these cases one gets two special points on the unit circle, instead of one, namely $\theta=0$ and $\theta=\pm\pi$, at which we have dominant singularities, which will be borderline hard singularities so long as $a_{j}\propto 1/j^{p}$ with $0<p\leq 1$.

In addition to this, series with step $1$ and coefficients that have alternating signs, such as $a_{k}=(-1)^{k}b_{k}$ with monotonic $b_{k}$, which are therefore not monotonic series, can be separated into two sub-series with step $2$, one with odd $k$ and the other with even $k$, and since the coefficients of these two sub-series are monotonic, then the result holds for each one of the two series, and hence for their sum. In this case we will have two dominant singularities in each one of the components series, located at $z=\pm 1$. However, sometimes the two singularities at $z=1$, one in each component series, may cancel off and the original series may have a single dominant singularity located at $z=-1$. One can see this by means of a simple transformation of variables,

$$(-1)^{k}b_{k}z^{k} = b_{k}z^{\prime k},$$

where $z'=-z$, so that $z'=1$ implies $z=-1$. Series with step $2$ and coefficients that have alternating signs, such as $a_{k}=(-1)^{j}b_{k}$ with $k=2j$ or $k=2j+1$ and monotonic $b_{k}$, which are also not monotonic themselves, can be separated into two sub-series with step $4$, and since the coefficients of these two sub-series are monotonic, then the result holds for each one of the two series, and hence for their sum. In this case we will have four dominant singularities in each one of the components series, located at $z=\pm 1$ and $z=\pm\mbox{\boldmath$\imath$}$. However, sometimes the singularities at $z=\pm 1$ of the two component series may cancel off and the original series may have only two dominant singularity located at $z=\pm\mbox{\boldmath$\imath$}$. One can see this by means of another simple transformation of variables,

$$(-1)^{j}b_{k}z^{2j} = b_{k}z^{\prime j},$$

where $z'=-z^{2}$, so that $z'=1$ implies $z^{2}=-1$ and hence $z=\pm\mbox{\boldmath$\imath$}$. In fact, the result can be generalized to series with non-zero terms only at some arbitrary regular interval $\Delta k$, that is, having non-zero terms with some constant step other than $2$. If we have a complex power series given by

$$S_{z} = \sum_{j=0}^{\infty} a_{k}z^{k},$$

where $k=k_{0}+pj$, for some strictly positive integer $k_{0}$ and where the step $p$ is another strictly positive integer, we may simply define new coefficients $a'_{j}=a_{k}$ and a new complex variable $z'=z^{p}$, in terms of which the series can now be written as

$$S_{z} = z^{k_{0}} \sum_{j=0}^{\infty} a'_{j}z^{\prime j},$$

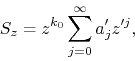
thus reducing it to the previous form, in terms of the variable $z'$. So long as the non-zero coefficients $a_{k}$ tend monotonically to zero as $k\to\infty$, we have that the coefficients $a'_{j}$ tend monotonically to zero as $j\to\infty$, and the previous result applies. In this case the special points over the unit circle are given by $z^{p}=1$, and there are, therefore, $p$ such points, including $z=1$, uniformly distributed along the circle. If combined with alternating signs, these series have special points given by $z^{p}=-1$, and once again there are $p$ such points uniformly distributed along the circle. Note that the number of dominant singularities on the unit circle increases with the step $p$, and that they are homogeneously distributed along that circle.

Finally, one may consider building finite superpositions of the series in all the previous cases discussed so far. Since each component series converges almost everywhere and has a finite number of dominant singularities, these superpositions of series will all converge, and will all have a finite number of dominant singularities on the unit disk. Since all the component series are of type $S_{v,h0}$, and hence have dominant singularities which are borderline hard ones, the dominant singularities of the superpositions will always be at most borderline hard ones. If the dominant singularities are in fact borderline hard ones, then we will call these series extended monotonic series. Each one of these series generates a different regular integral-differential chain, and this defines a rather large set of series that can be classified according to our scheme, relating their mode of convergence and the dominant singularities on the unit circle.