Another Regular Cosine Series with Odd $k$

Consider the Fourier series of the unit-amplitude square wave, shifted along the $\theta$ axis to $\theta'$, with $\theta-\theta'= \pi/2$, so that it becomes an even function. As is well known it is given by the cosine series

$$S_{\rm c} = \frac{4}{\pi} \sum_{j=0}^{\infty} \frac{(-1)^{j}}{2j+1}\, \cos[(2j+1)\theta],$$

where we have dropped the prime. The corresponding FC series is then

$$\bar{S}_{\rm c} = \frac{4}{\pi} \sum_{j=0}^{\infty} \frac{(-1)^{j}}{2j+1}\, \sin[(2j+1)\theta],$$

the complex $S_{v}$ series is given by

$$S_{v} = \frac{4}{\pi} \sum_{j=0}^{\infty} \frac{(-1)^{j}}{2j+1}\, v^{2j+1},$$

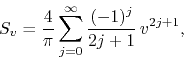
and the complex power series $S_{z}$ is given by

$$S_{z} = \frac{4}{\pi} \sum_{j=0}^{\infty} \frac{(-1)^{j}}{2j+1}\, z^{2j+1}.$$

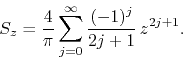
The ratio test tells us that the disk of convergence of $S_{z}$ is the unit disk. If we consider the inner analytic function $w(z)$ within this disk we observe that $w(0)=0$, as expected. We have for this function

$$w(z) = \frac{4}{\pi} \sum_{j=0}^{\infty} \frac{(-1)^{j}}{2j+1}\, z^{2j+1}.$$

Being given by a monotonic series of step $2$ modified by the factor of $(-1)^{j}$, this function has two dominant singularities, located at $z=\mbox{\boldmath$\imath$}$ and at $z=-\mbox{\boldmath$\imath$}$, where it diverges to infinity, as one can easily verify,

$$\begin{eqnarray*} w(\mbox{\boldmath$\imath$}) & = & \frac{4\mbox{\boldmath$\i... ... \frac{1}{2j+1} \\ & \to & -\mbox{\boldmath$\imath$}\infty. \end{eqnarray*}$$

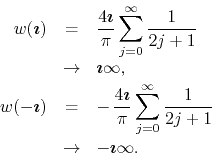
We must therefore use the two factors $(z-\mbox{\boldmath$\imath$})(z+\mbox{\boldmath$\imath$})=z^{2}+1$ in the construction of the center series,

$$\begin{eqnarray*} C_{z} & = & \frac{4}{\pi}\, \left(z^{2}+1\right) \sum_{j=... ...m_{j=1}^{\infty} \frac{2(-1)^{j}}{4j^{2}-1}\, z^{2j} \right]. \end{eqnarray*}$$

Unlike the original series, with coefficients that behave as $1/k$ (with $k=2j+1$), this series has coefficients that go to zero as $1/k^{2}$ when $k\to\infty$, and therefore is absolutely and uniformly convergent to a continuous function. This shows, in particular, that our evaluation of the set of dominant singularities of $w(z)$ was in fact correct. We have therefore for $S_{z}$ the representation

$$S_{z} = \frac{4}{\pi}\, \frac{z}{z^{2}+1} \left[ 1 - ... ...{j=1}^{\infty} \frac{2(-1)^{j}}{4j^{2}-1}\, z^{2j} \right],$$

with the singularities factored out and where the series involved is absolutely and uniformly convergent, and therefore converges much faster than the original one.

We may now take the real and imaginary parts of the $S_{v}$ series in order to obtain faster-converging representation of the original DP Fourier series and its FC series. We have on the unit circle

$$\begin{eqnarray*} \frac{z}{z^{2}+1} & = & \frac {z\left[(z^{*})^{2}+1\right]... ...theta)}{2\cos^{2}(\theta)} \\ & = & \frac{1}{2\cos(\theta)}, \end{eqnarray*}$$

and therefore

$$\begin{eqnarray*} S_{v} & = & \frac{2}{\pi\cos(\theta)} \left[ 1 - \sum_{... ...{\infty} \frac{2(-1)^{j}}{4j^{2}-1}\, \sin(2j\theta) \right]. \end{eqnarray*}$$

The original DP function is given by the real part,

$$f_{\rm c}(\theta) = \frac{2}{\pi\cos(\theta)} \left[ 1 ... ...infty} \frac{2(-1)^{j}}{4j^{2}-1}\, \cos(2j\theta) \right],$$

and the corresponding FC function $f_{\rm s}(\theta)=\bar{f}_{\rm c}(\theta)$ is given by the imaginary part,

$$f_{\rm s}(\theta) = -\, \frac{2}{\pi\cos(\theta)} \left... ...infty} \frac{2(-1)^{j}}{4j^{2}-1}\, \sin(2j\theta) \right].$$

Both of these series are absolutely and uniformly convergent.