Conclusions and Outlook

A very general proof of the existence of the solution of the Dirichlet boundary value problem of the Laplace equation on the plane was presented. The proof is valid not only for a very large class of real functions at the boundary, but also for a large class of boundary curves, with and without points of non-differentiability. The proof was presented in incremental steps, each generalizing the previous ones. The proofs for the unit circle are based on the complex-analytic structure within the unit disk presented and developed in previous papers [#!CAoRFI!#,#!CAoRFII!#,#!CAoRFIII!#,#!CAoRFIV!#]. The generalization for curves other than the unit circle uses the conformal mapping results associated to the famous Riemann mapping theorem. The most general statement of the theorem established here reads as follows.

$\parbox{\textwidth}{\bf Given a real function $f(\lambda)$\ that def... ...ior of $C$\ and that satisfies $u(x,y)=f(\lambda)$\ almost everywhere on $C$.}$

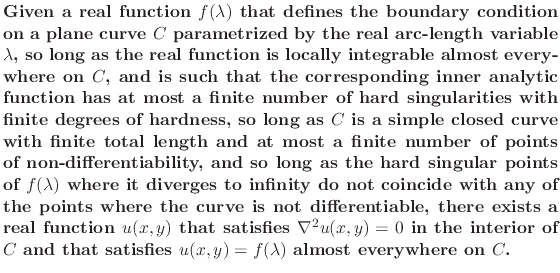
The proof is constructive, and consists of constructing from $f(\lambda)$ an analytic function in the interior of $C$, of which $u(x,y)$ is the real part. The theorem is quite general, including large classes of both boundary conditions and boundary curves.

Further extensions of the theorem may be possible. For example, the proofs established in Sections 2 and 3 can be rather trivially extended to include as well the whole space of singular Schwartz distributions discussed in [#!CAoRFII!#], that is, they can be extended to generalized real functions. This allows one to discuss some rather unusual Dirichlet problems in which the boundary condition is given by a singular real object such as the Dirac delta ``function'' or its derivatives. As mentioned in [#!CAoRFI!#], a possible further extension would be to real functions with a countable infinity of hard singular points which have, however, a finite number of accumulation points. The requirement that the hard singular points of $f(\lambda)$ where it diverges to infinity do not coincide with the points where the curve $C$ is non-differentiable seems to be a technical quirk, and probably can be eliminated. It is important to note that the proof is intrinsically limited to two-dimensional problems on the plane.

It is interesting to observe that the uniqueness of the solution can also be discussed in this context, in terms of the fact that $f(\theta)\equiv 0$ corresponds to the Fourier coefficients $\alpha_{0}=0$, $\alpha_{k}=0$ and $\beta_{k}=0$, for all $k$, and therefore to the complex Taylor coefficients $c_{0}=0$ and $c_{k}=0$, for all $k$, and therefore to the identically zero inner analytic function $w(z)\equiv 0$. Given an integrable real function $f(\theta)$ and two corresponding solutions $w_{1}(z)$ and $w_{2}(z)$ of the Dirichlet problem, we simply consider $w(z)=w_{2}(z)-w_{1}(z)$, which is therefore a solution of the Dirichlet problem with $f(\theta)\equiv 0$, and thus by construction is $w(z)\equiv 0$. It follows that $w_{2}(z)\equiv w_{1}(z)$, so that the solution is unique, in the sense that $u_{2}(\rho,\theta)=u_{1}(\rho,\theta)$ almost everywhere on the unit disk. We can say, in fact, that these two functions are equal at all points on the unit circle where they are well defined.

With some more work towards its generalization, the result presented here points, perhaps, to an even more general result, according to which the solution of the Dirichlet problem of the Laplace equation in two dimensions, in essence, always exists, in the sense that it exists under all conceivable circumstances in which it makes any sense at all to pose the corresponding boundary value problem. Already, even with the result as it is now, this is almost the case in what concerns the applications to Physics.