Introduction

In a previous paper [1] we have developed a correspondence between, on one side, the Fourier series and Fourier coefficients of real functions on the interval $[-\pi,\pi]$ and, on the other side, a complex analytic structure within the open unit disk, consisting of a set of inner analytic functions and their complex Taylor series. The reader is referred to that paper for the definition of many of the concepts and notations used in this one. In many cases this correspondence leads to the formulation of expressions involving modified trigonometric series that can converge very fast to a given real function, even when the Fourier series of that function diverges or converges very slowly, as shown in [2].

In order to establish the correspondence described above, in [1] we assumed that the Definite Parity (DP) real functions $f(\theta)$ under examination are such that their Fourier series and the corresponding Fourier Conjugate (FC) series converge together on at least one point on the interval $[-\pi,\pi]$, a domain which, in the context of the correspondence to be established, is mapped onto the unit circle of the complex plane. In this paper we will show that one can relax that hypothesis, replacing it by a much weaker one.

Following the development in [1], we will deal here only with real functions that have definite parity properties, which we will call Definite Parity real functions or DP real functions for short. Since any real function $f(\theta)$ in the interval $[-\pi,\pi]$, without any restriction, can be separated into even and odd parts,

$$\begin{eqnarray*} f(\theta) & = & f_{\rm c}(\theta)+f_{\rm s}(\theta), \\ ... ... \\ f_{\rm s}(\theta) & = & \frac{f(\theta)-f(-\theta)}{2}, \end{eqnarray*}$$

where

$$\begin{eqnarray*} f_{\rm c}(\theta) & = & f_{\rm c}(-\theta), \\ f_{\rm s}(\theta) & = & -f_{\rm s}(-\theta), \end{eqnarray*}$$

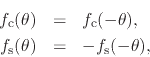
we can restrict the discussion to Definite Parity (DP) real functions without any loss of generality. This condition implies that the corresponding inner analytic function $w(z)$ has the property that it reduces to a real function on the interval $(-1,1)$ of the real axis, as discussed in [1]. For simplicity, we will also assume that $f(\theta)$ is a zero-average function, since adding a constant function to $f(\theta)$ is a trivial operation that does not significantly affect the issues under discussion here. This condition implies that the corresponding inner analytic function $w(z)$ has the property that $w(0)=0$, as discussed in [1].

In Subsection $8.1$ of [1] we have shown that, if there is any singularity of an analytic function $w(z)$ inside the open unit disk, then the sequence of Taylor-Fourier coefficients of its Taylor series diverges to infinity exponentially fast on the unit circle, as a function of the series index. In this paper we will discuss the converse of this statement. We will show here that the mere absence of an exponentially-fast divergence of the sequence of Fourier coefficients on the unit circle is enough to ensure the convergence of the corresponding complex power series inside the open unit disk, thus leading to the definition of a corresponding inner analytic function.

A note about the concept of integrability of real functions is in order at this point. What we mean by integrability in this paper is integrability in the sense of Lebesgue, with the use of the usual Lebesgue measure. We will assume that all the real functions at issue here are measurable in the Lebesgue measure. Therefore whenever we speak of real functions, it should be understood that we mean Lebesgue-measurable real functions. We will use the following result from the theory of measure and integration: for real functions defined within a compact interval, which are Lebesgue measurable, integrability and absolute integrability are equivalent conditions [3]. Therefore we will use the concepts of integrability and of absolute integrability interchangeably.