Next: Proof of Convergence to Up: Appendix: Technical Proofs Previous: Alternate Proof of the
As a curiosity, it is interesting to point out that a first-order linear
low-pass filter can be defined over a straight segment on the complex
plane. In this way a filter in the Cartesian coordinates of the complex
plane can be defined. Consider an analytic function ![]() anywhere on the
complex plane. Consider also a segment of length
anywhere on the
complex plane. Consider also a segment of length ![]() and a
fixed direction given by a constant angle
and a
fixed direction given by a constant angle ![]() with the real
axis. Given an arbitrary position
with the real
axis. Given an arbitrary position ![]() on the complex plane we define then
two other points by
on the complex plane we define then
two other points by
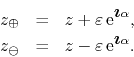
This defines a segment of length ![]() going from
going from ![]() to
to ![]() . Given any point
. Given any point ![]() such that this segment is contained
within the analyticity domain of
such that this segment is contained
within the analyticity domain of ![]() , we may now define a filtered
function
, we may now define a filtered
function
![]() by
by
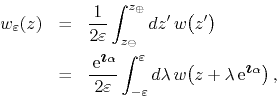
where ![]() is a real parameter describing the segment, such that
is a real parameter describing the segment, such that
![]() and
and
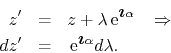
Since
![]() is analytic,
is analytic, ![]() and
and ![]() are
continuous, differentiable and satisfy the Cauchy-Riemann conditions in
Cartesian coordinates. We may write for
are
continuous, differentiable and satisfy the Cauchy-Riemann conditions in
Cartesian coordinates. We may write for
![]()
![\begin{eqnarray*}
w_{\varepsilon}(z)
& = &
u_{\varepsilon}(x,y)
+
\mbox{\bo...
...ilon}d\lambda\,
v[x+\lambda\cos(\alpha),y+\lambda\sin(\alpha)].
\end{eqnarray*}](img261.png)
It is now clear that
![]() and
and
![]() are
also continuous and differentiable. If we now take the partial derivatives
of these functions with respect to
are
also continuous and differentiable. If we now take the partial derivatives
of these functions with respect to ![]() we get
we get
![\begin{eqnarray*}
\frac{\partial u_{\varepsilon}}{\partial x}(x,y)
& = &
\fra...
...ial v}{\partial x}[x+\lambda\cos(\alpha),y+\lambda\sin(\alpha)].
\end{eqnarray*}](img264.png)
Using the Cauchy-Riemann conditions for ![]() and
and ![]() we get
we get
![\begin{eqnarray*}
\frac{\partial u_{\varepsilon}}{\partial x}(x,y)
& = &
\fra...
...ial v}{\partial x}[x+\lambda\cos(\alpha),y+\lambda\sin(\alpha)].
\end{eqnarray*}](img264.png)
Taking now the partial derivatives of
![]() and
and
![]() with respect to
with respect to ![]() we get
we get
![\begin{eqnarray*}
\frac{\partial u_{\varepsilon}}{\partial y}(x,y)
& = &
\fra...
...ial v}{\partial y}[x+\lambda\cos(\alpha),y+\lambda\sin(\alpha)].
\end{eqnarray*}](img266.png)
Comparing this pair of equation with the previous ones we finally get

This establishes that
![]() and
and
![]() satisfy the Cauchy-Riemann conditions, and therefore that
satisfy the Cauchy-Riemann conditions, and therefore that
![]() is analytic. Once
is analytic. Once
![]() is defined by
the filter at all points of the domain of analyticity of
is defined by
the filter at all points of the domain of analyticity of ![]() where the
segment fits, and now that it has been proven analytic there, one can
extend the definition of
where the
segment fits, and now that it has been proven analytic there, one can
extend the definition of
![]() to the whole domain of
analyticity of
to the whole domain of
analyticity of ![]() by analytic continuation.
by analytic continuation.