A Regular Cosine Series with Odd $k$

Consider the Fourier series of the unit-amplitude triangular wave. As is well known it is given by the cosine series

$$S_{\rm c} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, \cos[(2j+1)\theta].$$

The corresponding FC series is then

$$\bar{S}_{\rm c} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, \sin[(2j+1)\theta].$$

Note that due to the factors of $1/k^{2}$ (with $k=2j+1$), these series are already absolutely and uniformly convergent. But we will proceed with the construction in any case. The complex $S_{v}$ series is given by

$$S_{v} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, v^{2j+1},$$

and the complex power series $S_{z}$ is given by

$$S_{z} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, z^{2j+1}.$$

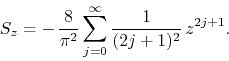
The ratio test tells us that the disk of convergence of $S_{z}$ is the unit disk. If we consider the inner analytic function $w(z)$ within this disk we observe that $w(0)=0$, as expected. We have for this function

$$w(z) = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{(2j+1)^{2}}\, z^{2j+1}.$$

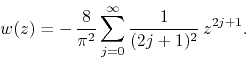
Being given by a monotonic series of step $2$ this function has two dominant singularities, located at $z=1$ and at $z=-1$, as one can easily verify by taking its logarithmic derivative, which is proportional to the inner analytic function of the square wave, that we examined before in Subsection B.2,

$$z\, \frac{dw(z)}{dz} = -\, \frac{8}{\pi^{2}} \sum_{j=0}^{\infty} \frac{1}{2j+1}\, z^{2j+1}.$$

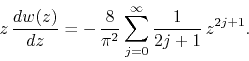
We must therefore use the two factors $(z-1)(z+1)=z^{2}-1$ in the construction of the center series,

$$\begin{eqnarray*} C_{z} & = & -\, \frac{8}{\pi^{2}}\, \left(z^{2}-1\right) ... ...infty} \frac{8j}{\left(4j^{2}-1\right)^{2}}\, z^{2j} \right]. \end{eqnarray*}$$

Unlike the original series, with coefficients that behave as $1/k^{2}$ (with $k=2j+1$), this series has coefficients that go to zero as $1/k^{3}$ when $k\to\infty$, and therefore converges faster than the original one. This shows, in particular, that our evaluation of the set of dominant singularities of $w(z)$ was in fact correct. We have therefore for $S_{z}$ the representation

$$S_{z} = \frac{8}{\pi^{2}}\, \frac{z}{z^{2}-1} \left[ 1... ...fty} \frac{8j}{\left(4j^{2}-1\right)^{2}}\, z^{2j} \right],$$

with the singularities factored out. Although both this series and the original one are absolutely and uniformly convergent, this converges faster, and may be differentiated once, still resulting in another series which is also absolutely and uniformly convergent. Note that in this case, as was discussed in the appendices of the previous paper [1], we are not able to write an explicit expression for $w(z)$ in terms of elementary function, so that we cannot explicitly take its limit to the unit circle. However, as one can see here we are still able to write a series to represent it over the unit circle, which converges faster that the original one. This gives us the possibility of calculating the function to any required precision level, and to do so efficiently.

We may now take the real and imaginary parts of the $S_{v}$ series in order to obtain faster-converging representation of the original DP Fourier series and its FC series. We have on the unit circle, as we saw before in Subsection B.2,

$$\frac{z}{z^{2}-1} = \frac{-\mbox{\boldmath$\imath$}}{2\sin(\theta)},$$

and therefore

$$\begin{eqnarray*} S_{v} & = & \frac{4}{\pi^{2}}\, \frac{-\mbox{\boldmath$\im... ...\frac{8j}{\left(4j^{2}-1\right)^{2}}\, \cos(2j\theta) \right]. \end{eqnarray*}$$

The original DP function is given by the real part,

$$f_{\rm c}(\theta) = -\, \frac{4}{\pi^{2}\sin(\theta)} \... ...rac{8j}{\left(4j^{2}-1\right)^{2}}\, \sin(2j\theta) \right],$$

and the corresponding FC function $f_{\rm s}(\theta)=\bar{f}_{\rm c}(\theta)$ is given by the imaginary part,

$$f_{\rm s}(\theta) = -\, \frac{4}{\pi^{2}\sin(\theta)} \... ...rac{8j}{\left(4j^{2}-1\right)^{2}}\, \cos(2j\theta) \right].$$

Both of these series converge faster than the original Fourier series.