Next: A Regular Sine Series Up: Appendix: Examples of Limits Previous: Appendix: Examples of Limits
Consider the Fourier series of the one-cycle unit-amplitude sawtooth wave,
which is just the linear function ![]() between
between ![]() and
and ![]() .
As is well known it is given by the sine series
.
As is well known it is given by the sine series
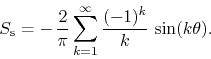
The corresponding FC series is then
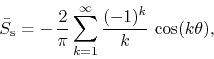
the complex ![]() series is given by
series is given by
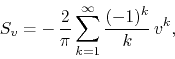
and the complex power series ![]() is given by
is given by
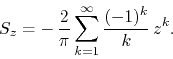
The ratio test tells us that the disk of convergence of ![]() is the
unit disk. If we consider the inner analytic function
is the
unit disk. If we consider the inner analytic function ![]() within this
disk we observe that
within this
disk we observe that ![]() , as expected. We have for the function and
its derivative
, as expected. We have for the function and
its derivative
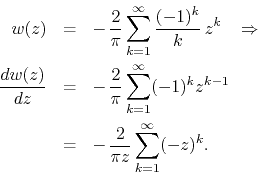
This is a geometrical series and therefore we may write the derivative in closed form,
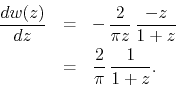
This function has a simple pole at the point where the one-cycle sawtooth
wave is discontinuous. This is a hard singularity of degree ![]() . It is now
very simple to integrate in order to obtain the inner analytic function
. It is now
very simple to integrate in order to obtain the inner analytic function
![]() in closed form, remembering that we should have
in closed form, remembering that we should have ![]() ,
,
This function has a logarithmic singularity at the point where the one-cycle sawtooth wave is discontinuous. This is a borderline hard singularity. If we now rationalize the argument of the logarithm in order to write it in the form
then the logarithm is given by
which allows us to identify the real and imaginary parts of ![]() . We may
write for the argument
. We may
write for the argument
![\begin{eqnarray*}
R\,{\rm e}^{\mbox{\boldmath$\imath$}\alpha}
& = &
1
+
\rh...
...
\mbox{\boldmath$\imath$}
\left[
\rho
\sin(\theta)
\right].
\end{eqnarray*}](img277.png)
From this it follows, after some algebra, that we have
![\begin{eqnarray*}
R(\rho,\theta)
& = &
\sqrt{1+2\rho\cos(\theta)+\rho^{2}},
...
...tan\!
\left[\frac{\rho\sin(\theta)}{1+\rho\cos(\theta)}\right].
\end{eqnarray*}](img278.png)
The part which is of interest now is the imaginary one, which is related
to the series ![]() ,
,
The ![]() limit of this quantity is the function
limit of this quantity is the function
![]() . If we consider the case
. If we consider the case ![]() , we immediately get
zero, because the argument of the arc tangent is zero, and therefore we
get
, we immediately get
zero, because the argument of the arc tangent is zero, and therefore we
get ![]() . Therefore we have
. Therefore we have
![\begin{eqnarray*}
f_{\rm s}(\pm\pi)
& = &
\lim_{\rho\to 1}
\Im[w(\rho,\pm\pi)]
\\
& = &
0.
\end{eqnarray*}](img281.png)
This is the correct value for the Fourier-series representation of the
one-cycle sawtooth wave at this point. For
![]() we get
we get
![\begin{eqnarray*}
f_{\rm s}(\theta)
& = &
\lim_{\rho\to 1}
\Im[w(z)]
\\
&...
...i}
\arctan\!
\left[\frac{\sin(\theta)}{1+\cos(\theta)}\right].
\end{eqnarray*}](img283.png)
In order to solve this we write everything in terms of ![]() , and
thus obtain
, and
thus obtain
![\begin{eqnarray*}
f_{\rm s}(\theta)
& = &
\frac{2}{\pi}
\arctan\!
\left[\fr...
...an\!\left[\tan(\theta/2)\right]
\\
& = &
\frac{\theta}{\pi}.
\end{eqnarray*}](img285.png)
This is the correct value for the one-cycle unit-amplitude sawtooth wave. The FC function is related to the real part
In the ![]() limit this function is singular at
limit this function is singular at ![]() .
Away from this logarithmic singularity we may write
.
Away from this logarithmic singularity we may write
![\begin{eqnarray*}
\bar{f}_{\rm s}(\theta)
& = &
\lim_{\rho\to 1}
\Re[w(z)]
...
...
& = &
\frac{1}{\pi}\,
\ln\!\left[4\cos^{2}(\theta/2)\right].
\end{eqnarray*}](img287.png)
We see therefore that the FC function to the one-cycle sawtooth wave is a function with a logarithmic singularity at the point where the original function is discontinuous. The derivative of this conjugate function is easily calculated, and turns out to be
This is a quite a simple function, with a simple pole at ![]() .
Note that the logarithmic singularity of the FC function
.
Note that the logarithmic singularity of the FC function
![]() is an integrable one, as one would expect since the Fourier
coefficients are finite.
is an integrable one, as one would expect since the Fourier
coefficients are finite.
Note that we get a free set of integration formulas out of this effort.
Since the coefficients ![]() can be written as integrals involving
can be written as integrals involving
![]() , we have at once that
, we have at once that
![\begin{displaymath}
\int_{-\pi}^{\pi}d\theta\,
\ln\!\left[4\cos^{2}(\theta/2)\right]
\cos(k\theta)
=
-\,
\frac{2\pi(-1)^{k}}{k},
\end{displaymath}](img289.png)
for ![]() .
.